hexagonal grid of resistors

On this infinite hexagonal grid of ideal one-ohm resistors, what’s the equivalent resistance between the two marked nodes?

This is a variation of the following xkcd:

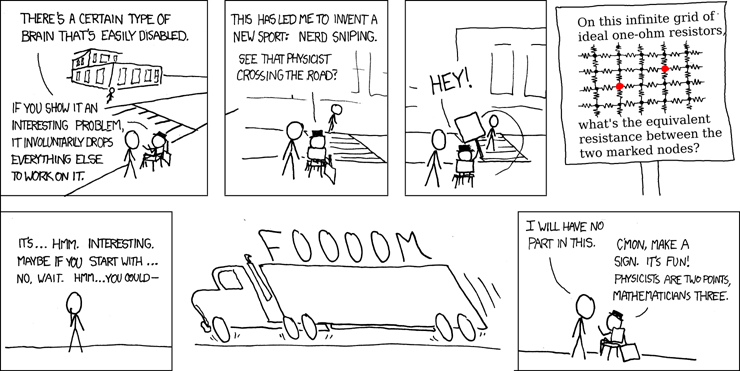
Of which the solution can be found here: https://www.mathpages.com/home/kmath668/kmath668.htm

As this is already a really difficult problem, one might assume that a hexagonal grid makes this problem even harder. And in general, this obviously does, but these two marked points are close enough together that we can use the symmetries of the system to calculate the resistance.

First, let’s use the same method and assumptions of the system in the provided solution. We apply a current of 1 Amp to one of the nodes, and a current of -1 Amp to the other node, and calculate the potential difference between them. Due to the linearity of the system, one can use the Superposition principle to only apply a single current at one of the nodes, and use the symmetry to state that applying the other one will cause the same difference in potential. So we only have to solve the potential difference between our two nodes, given that there is an external current of 1 Amp in one marked node, and one of zero everywhere. Our solution will then be twice this potential difference.

If we apply a current of 1 Amp to a node, the current will flow out as follows:

The currents marked in blue are all equal, so are the currents marked in green, due to the symmetry of the system. ## Kirchhoff’s current law tells us that the current over in the blue arrows is $1/3A$ and that the current over the green arrows is $1/6A$. This also equals the voltage over this resistor, as all resistances are $1\Omega$. Thus our effective resistance between the two nodes is
$2 \left(\frac{1}{3}+\frac{1}{6}\right)$
Which is equal to 1. So our effective resistance is $1\Omega$